Month: August 2023

Researchers are using mathematical models to better understand the effects of disruptions like daylight savings time, working night shifts, jet lag or even late-night phone scrolling on the body’s circadian rhythms.

The University of Waterloo and the University of Oxford researchers have developed a new model to help scientists better understand the resilience of the brain’s master clock: the cluster of neurons in the brain that coordinates the body’s other internal rhythms. They also hope to suggest ways to help improve this resilience in individuals with weak or impaired circadian rhythms. The study, “Can the Clocks Tick Together Despite the Noise? Stochastic Simulations and Analysis,” appears in the SIAM Journal on Applied Dynamical Systems.

Sustained disruptions to circadian rhythm have been linked to diabetes, memory loss, and many other disorders.

“Current society is experiencing a rapid increase in demand for work outside of traditional daylight hours,” said Stéphanie Abo, a Ph.D. student in applied mathematics and the study’s lead author. “This greatly disrupts how we are exposed to light, as well as other habits such as eating and sleeping patterns.”

Humans’ circadian rhythms, or internal clocks, are the roughly 24-hour cycles many body systems follow, usually alternating between wakefulness and rest. Scientists are still working to understand the cluster of neurons known as suprachiasmatic nucleus (SCN) or master clock.

Using mathematical modeling techniques and differential equations, the team of applied mathematics researchers modeled the SCN as a macroscopic, or big-picture, system comprised of a seemingly infinite number of neurons. They were especially interested in understanding the system’s couplings—the connections between neurons in the SCN that allow it to achieve a shared rhythm.

Frequent and sustained disturbances to the body’s circadian rhythms eliminated the shared rhythm, implying a weakening of the signals transmitted between SCN neurons.

Abo said they were surprised to find that “a small enough disruption can actually make the connections between neurons stronger.”

“Mathematical models allow you to manipulate body systems with specificity that cannot be easily or ethically achieved in the body or a petri dish,” Abo said. “This allows us to do research and develop good hypotheses at a lower cost.”

For more such insights, log into our website https://international-maths-challenge.com

Credit of the article given to University of Waterloo

Graphic novels can help make math and physics more accessible for students, parents or teachers in training. Metamorworks/iStock via Getty Images

Post-pandemic, some educators are trying to reengage students with technology – like videos, computer gaming or artificial intelligence, just to name a few. But integrating these approaches in the classroom can be an uphill battle. Teachers using these tools often struggle to retain students’ attention, competing with the latest social media phenomenon, and can feel limited by using short video clips to get concepts across.

Graphic novels – offering visual information married with text – provide a means to engage students without losing all of the rigor of textbooks. As two educators in math and physics, we have found graphic novels to be effective at teaching students of all ability levels. We’ve used graphic novels in our own classes, and we’ve also inspired and encouraged other teachers to use them. And we’re not alone: Other teachers are rejuvenating this analog medium with a high level of success.

In addition to covering a wide range of topics and audiences, graphic novels can explain tough topics without alienating student averse to STEM – science, technology, engineering and math. Even for students who already like math and physics, graphic novels provide a way to dive into topics beyond what is possible in a time-constrained class. In our book “Using Graphic Novels in the STEM Classroom,” we discuss the many reasons why graphic novels have a unique place in math and physics education. Here are three of those reasons:

Explaining complex concepts with rigor and fun

Increasingly, schools are moving away from textbooks, even though studies show that students learn better using print rather than digital formats. Graphic novels offer the best of both worlds: a hybrid between modern and traditional media.

This integration of text with images and diagrams is especially useful in STEM disciplines that require quantitative reading and data analysis skills, like math and physics.

For example, our collaborator Jason Ho, an assistant professor at Dordt University, uses “Max the Demon Vs Entropy of Doom” to teach his physics students about entropy. This topic can be particularly difficult for students because it’s one of the first times when they can’t physically touch something in physics. Instead, students have to rely on math and diagrams to fill in their knowledge.

Rather than stressing over equations, Ho’s students focus on understanding the subject more conceptually. This approach helps build their intuition before diving into the algebra. They get a feeling for the fundamentals before they have to worry about equations.

After having taken Ho’s class, more than 85% of his students agreed that they would recommend using graphic novels in STEM classes, and 90% found this particular use of “Max the Demon” helpful for their learning. When strategically used, graphic novels can create a dynamic, engaging teaching environment even with nuanced, quantitative topics.

Combating quantitative anxiety

Students learning math and physics today are surrounded by math anxiety and trauma, which often lead to their own negative associations with math. A student’s perception of math can be influenced by the attitudes of the role models around them – whether it’s a parent who is “not a math person” or a teacher with a high level of math anxiety.

Graphic novels can help make math more accessible not only for students themselves, but also for parents or students learning to be teachers.

In a geometry course one of us (Sarah) teaches, secondary education students don’t memorize formulas and fill out problem sheets. Instead, students read “Who Killed Professor X?”, a murder mystery in which all of the suspects are famous mathematicians. The suspects’ alibis are justified through problems from geometry, algebra and pre-calculus.

While trying to understand the hidden geometry of suspect relationships, students often forget that they are doing math – focusing instead on poring over secret hints and notes needed to solve the mystery.

Although this is just one experience for these students, it can help change the narrative for students experiencing mathematical anxiety. It boosts their confidence and shows them how math can be fun – a lesson they can then impart to the next generation of students.

Helping students learn and readers dream big

In addition to being viewed favourably by students, graphic novels can enhance student learning by improving written communication skills, reading comprehension and critical literacy skills. And even outside the classroom, graphic novels support long-term memory for those who have diagnoses like dyslexia.

Pause and think about your own experience – how do you learn about something new in science?

If you’re handed a textbook, it’s extremely unlikely that you’d read it cover to cover. And although the internet offers an enormous amount of math and physics content, it can be overwhelming to sift through hours and hours of videos to find the perfect one to get the “aha!” moment in learning.

Graphic novels provide a starting point for such a broad range of niche topics that it’s impossible for anyone to be experts in them all. Want to learn about programming? Try the “Secret Coders” series. Want to understand more about quantum physics? Dive into “Suspended in Language: Niels Bohr’s life, discoveries, and the century he shaped.” Searching for more female role models in science? “Astronauts: Women on the Final Frontier” could be just what you’re looking for.

With all that they offer, graphic novels provide a compelling list of topics and narratives that can capture the attention of students today. We believe that the right set of graphic novels can inspire the next generation of scientists as much as any single individual can.

For more such insights, log into our website https://international-maths-challenge.com

From avoiding the number seven to picking numbers over 31, mathematician Peter Rowlett has a few psychological strategies for improving your chances when playing the lottery.

Would you think I was daft if I bought a lottery ticket for the numbers 1, 2, 3, 4, 5 and 6? There is no way those are going to be drawn, right? That feeling should – and, mathematically, does – actually apply to any set of six numbers you could pick.

Lotteries are ancient. Emperor Augustus, for example, organised one to fund repairs to Rome. Early lotteries involved selling tickets and drawing lots, but the idea of people guessing which numbers would be drawn from a machine comes from Renaissance Genoa. A common format is a game that draws six balls from 49, studied by mathematician Leonhard Euler in the 18th century.

The probabilities Euler investigated are found by counting the number of possible draws. There are 49 balls that could be drawn first. For each of these, there are 48 balls that can be drawn next, so there are 49×48 ways to draw two balls. This continues, so there are 49×48×47×46×45×44 ways to draw six balls. But this number counts all the different arrangements of any six balls as a unique solution.

How many ways can we rearrange six balls? Well, we have six choices for which to put first, then for each of these, five choices for which to put second, and so on. So the number of ways of arranging six balls is 6×5×4×3×2×1, a number called 6! (six factorial). We divide 49×48×47×46×45×44 by 6! to get 13,983,816, so the odds of a win are near 1 in 14 million.

Since all combinations of numbers are equally likely, how can you maximise your winnings? Here is where maths meets psychology: you win more if fewer people share the prize, so choose numbers others don’t. Because people often use dates, numbers over 31 are chosen less often, as well as “unlucky” numbers like 13. A lot of people think of 7 as their favourite number, so perhaps avoid it. People tend to avoid patterns so are less likely to pick consecutive or regularly spaced numbers as they feel less random.

In July, David Cushing and David Stewart at the University of Manchester, UK, published a list of 27 lottery tickets that guarantee a win in the UK National Lottery, which uses 59 balls and offers a prize for matching two or more. But a win doesn’t always mean a profit – for almost 99 per cent of possible draws, their tickets match at most three balls, earning prizes that may not exceed the cost of the tickets!

So, is a lottery worth playing? Since less than half the proceeds are given out in prizes, you would probably be better off saving your weekly ticket money. But a lecturer of mine made an interesting cost-benefit argument. He was paid enough that he could lose the cost of a ticket each week without really noticing. But if he won the jackpot, his life would be changed. So, given that lottery profit is often used to support charitable causes, it might just be worth splurging.

For more such insights, log into www.international-maths-challenge.com.

*Credit for article given to Peter Rowlett*

Life’s random rhythms surround us–from the hypnotic, synchronized blinking of fireflies…to the back-and-forth motion of a child’s swing… to slight variations in the otherwise steady lub-dub of the human heart.

But truly understanding those rhythms—called stochastic, or random, oscillations—has eluded scientists. While researchers and clinicians have some success in parsing brain waves and heartbeats, they’ve been unable to compare or catalog an untold number of variations and sources.

Gaining such insight into the underlying source of oscillations “could lead to advances in neural science, cardiac science and any number of different fields,” said Peter Thomas, a professor of applied mathematics at Case Western Reserve University.

Thomas is part of an international team that says it has developed a novel, universal framework for comparing and contrasting oscillations—regardless of their different underlying mechanisms—which could become a critical step toward someday fully understanding them.

Their findings were recently published in Proceedings of the National Academy of Sciences.

“We turned the problem of comparing oscillators into a linear algebra problem,” Thomas said. “What we have done is vastly more precise than what was available before. It’s a major conceptual advance.”

The researchers say others can now compare, better understand—and even manipulate—oscillators previously considered to have completely different properties.

“If your heart cells aren’t synchronized, you die of atrial fibrillation,” Thomas said. “But if your brain cells synchronize too much, you have Parkinson’s disease, or epilepsy, depending on which part of the brain the synchronization occurs in. By using our new framework, that heart or brain scientist may be able to better understand what the oscillations could mean and how the heart or brain is working or changing over time.”

Swaying skyscrapers and brain waves

Thomas said the researchers—who included collaborators from universities in France, Germany and Spain—found a new way to use complex numbers to describe the timing of oscillators and how “noisy,” or imprecisely timed, they are.

Most oscillations are irregular to some extent, Thomas said. For example, a heart rhythm is not 100% regular. A natural variation of 5-10% in the heartbeat is considered healthy.

Thomas said the problem with comparing oscillators can be illustrated by considering two markedly different examples: brain rhythms and swaying skyscrapers.

“In San Francisco, modern skyscrapers sway in the wind, buffeted by randomly shifting air currents—they’re pushed slightly out of their vertical posture, but the mechanical properties of the structure pull them back,” he said. “This combination of flexibility and resilience helps high-rise buildings survive shaking during earthquakes. You wouldn’t think this process could be compared with brain waves, but our new formalism lets you compare them.”

How their findings might help either discipline—mechanical engineering and neuroscience—may be unknown right now, Thomas said, comparing the conceptual advance to when Galileo discovered Jupiter’s orbiting moons.

“What Galileo realized was a new point of view, and while our discovery is not as far-reaching as Galileo’s, it is similarly a change in perspective,” he said. “What we report in our paper is an entirely new point of view on stochastic oscillators.”

For more such insights, log into our website https://international-maths-challenge.com

Credit of the article given to Case Western Reserve University

Everyone knows that arithmetic is true: 2 + 2 = 4.

But surprisingly, we don’t know why it’s true.

By stepping outside the box of our usual way of thinking about numbers, my colleagues and I have recently shown that arithmetic has biological roots and is a natural consequence of how perception of the world around us is organized.

Our results explain why arithmetic is true and suggest that mathematics is a realization in symbols of the fundamental nature and creativity of the mind.

Thus, the miraculous correspondence between mathematics and physical reality that has been a source of wonder from the ancient Greeks to the present—as explored in astrophysicist Mario Livio’s book “Is God a Mathematician?”—suggests the mind and world are part of a common unity.

Why is arithmetic universally true?

Humans have been making symbols for numbers for more than 5,500 years. More than 100 distinct notation systems are known to have been used by different civilizations, including Babylonian, Egyptian, Etruscan, Mayan and Khmer.

The remarkable fact is that despite the great diversity of symbols and cultures, all are based on addition and multiplication. For example, in our familiar Hindu-Arabic numerals: 1,434 = (1 x 1000) + (4 x 100) + (3 x 10) + (4 x 1).

Why have humans invented the same arithmetic, over and over again? Could arithmetic be a universal truth waiting to be discovered?

To unravel the mystery, we need to ask why addition and multiplication are its fundamental operations. We recently posed this question and found that no satisfactory answer—one that met standards of scientific rigor—was available from philosophy, mathematics or the cognitive sciences.

The fact that we don’t know why arithmetic is true is a critical gap in our knowledge. Arithmetic is the foundation for higher mathematics, which is indispensable for science.

Consider a thought experiment. Physicists in the future have achieved the goal of a “theory of everything” or “God equation.” Even if such a theory could correctly predict all physical phenomena in the universe, it would not be able to explain where arithmetic itself comes from or why it is universally true.

Answering these questions is necessary for us to fully understand the role of mathematics in science.

Bees provide a clue

We proposed a new approach based on the assumption that arithmetic has a biological origin.

Many non-human species, including insects, show an ability for spatial navigation which seems to require the equivalent of algebraic computation. For example, bees can take a meandering journey to find nectar but then return by the most direct route, as if they can calculate the direction and distance home.

How their miniature brain (about 960,000 neurons) achieves this is unknown. These calculations might be the non-symbolic precursors of addition and multiplication, honed by natural selection as the optimal solution for navigation.

Arithmetic may be based on biology and special in some way because of evolution’s fine-tuning.

Stepping outside the box

To probe more deeply into arithmetic, we need to go beyond our habitual, concrete understanding and think in more general and abstract terms. Arithmetic consists of a set of elements and operations that combine two elements to give another element.

In the universe of possibilities, why are the elements represented as numbers and the operations as addition and multiplication? This is a meta-mathematical question—a question about mathematics itself that can be addressed using mathematical methods.

In our research, we proved that four assumptions—monotonicity, convexity, continuity and isomorphism—were sufficient to uniquely identify arithmetic (addition and multiplication over the real numbers) from the universe of possibilities.

• Monotonicityis the intuition of “order preserving” and helps us keep track of our place in the world, so that when we approach an object it looms larger but smaller when we move away.
• Convexityis grounded in intuitions of “betweenness.” For example, the four corners of a football pitch define the playing field even without boundary lines connecting them.
• Continuitydescribes the smoothness with which objects seem to move in space and time.
• Isomorphismis the idea of sameness or analogy. It’s what allows us to recognize that a cat is more similar to a dog than to a rock.

Thus, arithmetic is special because it is a consequence of these purely qualitative conditions. We argue that these conditions are principles of perceptual organization that shape how we and other animals experience the world—a kind of “deep structure” in perception with roots in evolutionary history.

In our proof, they act as constraints to eliminate all possibilities except arithmetic—a bit like how a sculptor’s work reveals a statue hidden in a block of stone.

What is mathematics?

Taken together, these four principles structure our perception of the world so that our experience is ordered and cognitively manageable. They are like colored spectacles that shape and constrain our experience in particular ways.

When we peer through these spectacles at the abstract universe of possibilities, we “see” numbers and arithmetic.

Thus, our results show that arithmetic is biologically based and a natural consequence of how our perception is structured.

Although this structure is shared with other animals, only humans have invented mathematics. It is humanity’s most intimate creation, a realization in symbols of the fundamental nature and creativity of the mind.

In this sense, mathematics is both invented (uniquely human) and discovered (biologically based). The seemingly miraculous success of mathematics in the physical sciences hints that our mind and the world are not separate, but part of a common unity.

The arc of mathematics and science points toward nondualism, a philosophical concept that describes how the mind and the universe as a whole are connected, and that any sense of separation is an illusion. This is consistent with many spiritual traditions (Taoism, Buddhism) and Indigenous knowledge systems such as mātauranga Māori.

For more such insights, log into our website https://international-maths-challenge.com

Credit of the article given to Randolph Grace, The Conversation

A team of physicists and mathematicians at the Institute for Basic Science’s Center for Soft and Living Matter, in South Korea, working with a colleague from the University of Geneva, has developed an algorithm that can be used to find the shape of an object to cause it to roll down a ramp following a desired path.

In their paper published in the journal Nature, the group describes how they developed their algorithm, and possible uses for it. Elisabetta Matsumoto and Henry Segerman with the Georgia Institute of Technology and Oklahoma State University, respectively, have published a News & Views piece in the same journal issue outlining the work done by the team on this new effort.

In this new effort, the research team started with an interesting puzzle—one that begins by envisioning a sphere rolling down a ramp. If the sphere is imagined to be made of clay, it can be manipulated (deformed) as it rolls to make it conform to a given path.

If the sphere is then rolled down the ramp again, it will follow the previous path due to the new deformities in its shape. The researchers noted that the paths that could be taken by the sphere could be nearly limitless due to the nearly limitless possible deformations.

That realization led them to wonder if the deformations that form in such a sphere could be corelated mathematically with its path. And if so, if such math could be used to create an algorithm that could be used to 3D print a sphere with deformations that would force it to follow a predetermined path.

It turned out the answer to both questions was yes. The team used math and physics principles to create formulas that described deformations to a given object that would result in the object following a desired path down an inclined plane. They then created a computer program that could be used to create such an object in the real world, using 3D printing.

The team named the objects trajectoids. Each had a solid metal ball-bearing inside to give it weight. They also found that they could create trajectoids that traveled over a given path twice, and named them “two-period trajectoids.”

The research team suggests their formulas and algorithm could be used in robotics applications and also in physics research associated with the angular moment of an electron—or in quantum research centered around the study of evolution of a quantum bit.

For more such insights, log into our website https://international-maths-challenge.com

Credit of the article given to Bob Yirka , Phys.org

Terry Loring, distinguished professor of mathematics and statistics, published and co-authored a new research piece involving his research on K-theory with the major advances in applications to critical problems in physics.

The study titled, “Revealing topology in metals using experimental protocols inspired by K-theory,” was published in Nature Communications. Loring used mathematical properties of K-theory to help advance the understanding of topological materials in the physics world.

The main focus of the study was to discover how electricity, sound, or light can be trapped in a portion of a material. “This experiment was done in what is called a meta-material, built from individual sound resonators coupled in a fashion that mimics how atoms can come together to form a crystal. Three-dimensional printing allows us to make customized resonators that we join in a precise way to make the physics match the mathematics,” explained Loring. The study was part of a larger project that covered many areas of physics.

According to Loring, there are different forms of K-theory that arise in many different mathematical fields, however, the form of K-theory that he used in this study was focused on being best suited for studying matrix models of physical systems.

Loring explains that matrices are simply square tables of numbers, with a peculiar rule for how two matrices are multiplied. This rule has an asymmetry in it that leads to having AB and BA sometimes being very different, meaning that the commutative law for multiplication is violated.

“Physicists like Heisenberg realized that matrices are terrific at modeling uncertainty in molecular- and atomic-scale physics. K-theory can tell us when certain matrices can be connected except by a path that goes through what we call a singular matrix. This guaranteed singularity turns out to have an important meaning when the matrices come from models of physical systems,” Loring said.

The researchers were mainly looking at topological materials which include topological insulators. A topological insulator can have an index associated to it, which is a number computed using K-theory. If a device is built from two topological insulators that each have a different index, there is guaranteed to be a conducting region where the two materials come together.

“This conducting region exactly corresponds to where a certain matrix goes singular. To demonstrate this fact we use results about determinants one learns in linear algebratogether with the intermediate value theorem that people learn in their first calculus class,” said Loring.

This research is attempting to advance the theory of topological metals. Topological metals mix up conducting and insulating properties in very confusing ways. Loring and team built an acoustic crystal that had a specific pattern, they then deliberately broke the pattern in the middle thus inserting a defect in the system.

“During the experiment, and computer simulations, we were able to show how sound can get trapped at the defect. The hope is that it teaches us how to better trap light in small-scale photonic devices, and more generally start to manipulate light in a similar way to how electronic circuits manipulate electricity. There are advantages to moving information with light, as this can sometimes eliminate/reduce the energy wasted by the heat associated with electronics,” Loring stated.

Another part of the experiment which was more delicate included modifying the acoustic resonators by a formula from K-theory. The modified system removed the metallic properties in many parts of the crystal, isolating the binding metallic nature of the defect.

“Of course our acoustics system is not a metal, but shares mathematical properties with metals that harbor topology in their electronic structure. The hope is we will be able to devise experimental probes of photonic and electronic systems that bring the K-theory off the blackboard and into the lab,” explained Loring.

Mathematics was central to the design of this experiment. The project began with a discussion of formulas in K-theory that might lead to a matrix that can describe the energy in an acoustic system.

“We started with the analysis we would use to explain the system and then built a system that could be analysed this way. This backwards flow is somewhat common in the field of ‘topological physics’ where clean formulas in math suggest the search for physical systems that match that formula,” Loring stated.

In finding, Loring and his team discovered that new mathematics can classify very local patches of material as insulating or conducting. Loring points out that initially it was not clear if this classification had any meaning that a physicist would care about.

“This experiment showed that we can manufacture materials where this local classification is physically meaningful. While this material has no practical application, it is expected that materials and devices will be discovered or manufactured that have these local variations and that these local variations will give us even more control over light and electricity than we now enjoy.”

For more such insights, log into our website https://international-maths-challenge.com

Credit of the article given to Dani Rae Wascher, University of New Mexico

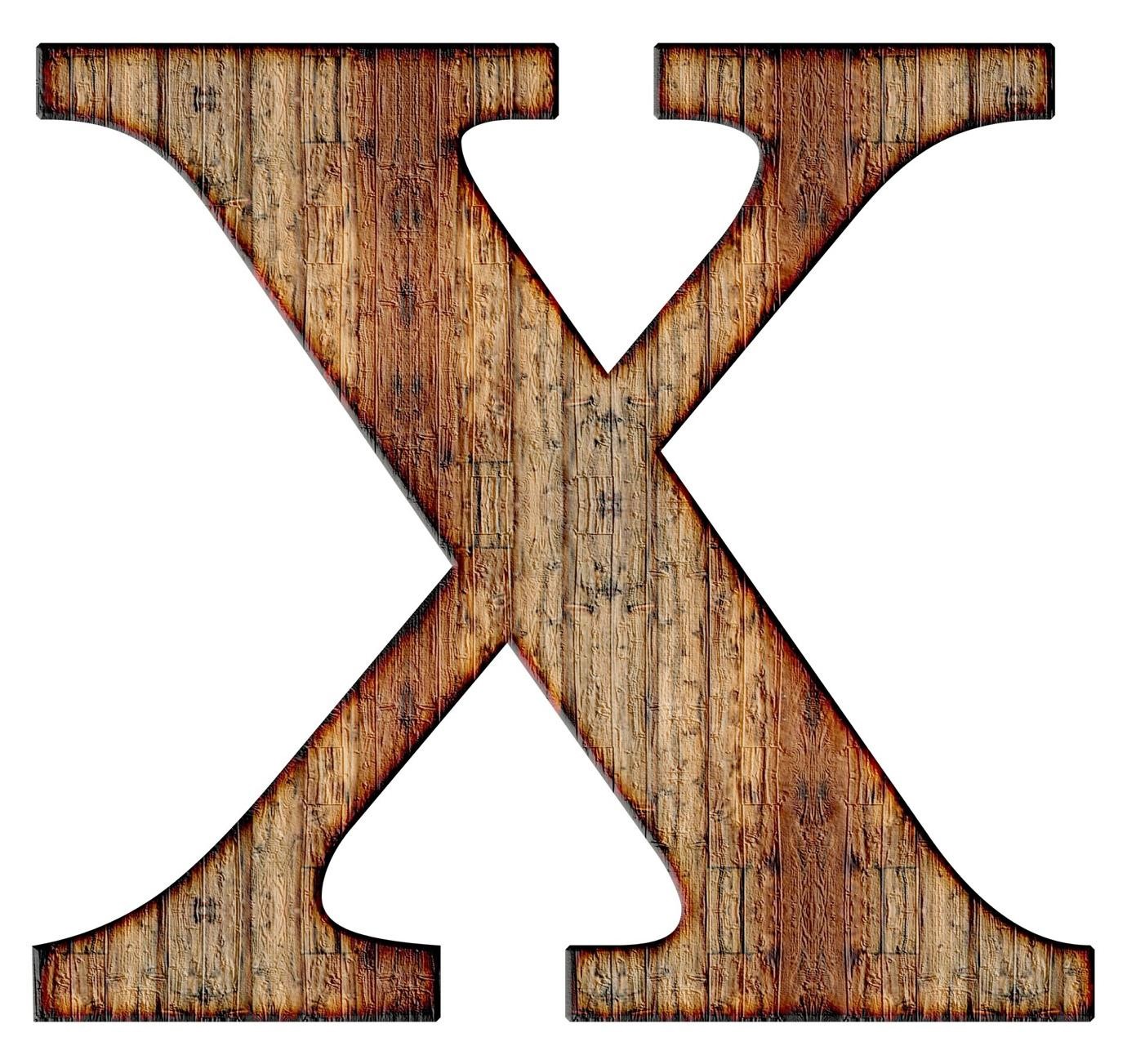
Even though “x” is one of the least-used letters in the English alphabet, it appears throughout American culture—from Stan Lee’s X-Men superheroes to “The X-Files” TV series. The letter x often symbolizes something unknown, with an air of mystery that can be appealing—just look at Elon Musk with SpaceX, Tesla’s Model X, and now X as a new name for Twitter.

You might be most familiar with x from math class. Many algebra problems use x as a variable, to stand in for an unknown quantity. But why is x the letter chosen for this role? When and where did this convention begin?

There are a few different explanations that math enthusiasts have put forward—some citing translation, others pointing to a more typographic origin. Each theory has some merit, but historians of mathematics, like me, know that it’s difficult to say for sure how x got its role in modern algebra.

Ancient unknowns

Algebra today is a branch of math in which abstract symbols are manipulated, using arithmetic, to solve different kinds of equations. But many ancient societies had well-developed mathematical systems and knowledge with no symbolic notation.

All ancient algebra was rhetorical. Mathematical problems and solutions were completely written out in words as part of a little story, much like the word problems you might see in elementary school.

Ancient Egyptian mathematicians, who are perhaps best known for their geometric advances, were skilled in solving simple algebraic problems. In the Rhind papyrus, the scribe Ahmes uses the hieroglyphics referred to as “aha” to denote the unknown quantity in his algebraic problems. For example, problem 24 asks for the value of aha if aha plus one-seventh of aha equals 19. “Aha” means something like “mass” or “heap.”

The ancient Babylonians of Mesopotamia used many different words for unknowns in their algebraic system—typically words meaning length, width, area or volume, even if the problem itself was not geometric in nature. One ancient problem involved two unknowns termed the “first silver thing” and the “second silver thing.”

Mathematical know-how developed somewhat independently in many lands and in many languages. Limitations in communication prevented any immediate standardization of notation. However, over time some abbreviations crept in.

In a transitional syncopated phase, authors used some symbolic notation, but algebraic ideas were still presented mainly rhetorically. Diophantus of Alexandria used a syncopated algebra in his great work Arithmetica. He called the unknown “arithmos” and used an archaic Greek letter similar to s for the unknown.

Indian mathematicians made additional algebraic discoveries and developed what are essentially the modern symbols for each of the decimal digits. One especially influential Indian mathematician was Brahmagupta, whose algebraic techniques could handle any quadratic equation. Brahmagupta’s name for the unknown variable was yãvattâvat. When additional variables were required, he instead used the initial syllable of color names, like kâ from kâlaka (black), ya from yavat tava (yellow), ni from nilaka (blue), and so on.

Islamic scholars translated and preserved a great deal of both Greek and Indian scholarship that has contributed immensely to the world’s mathematical, scientific and technical knowledge. The most famous Islamic mathematician was al-Khowarizmi, whose foundational book Al-jabr wa’l muqabalah is at the root of the modern word “algebra.”

So what about x?

One theory of the genesis of x as the unknown in modern algebra points to these Islamic roots. The theory contends that the Arabic word used for the quantity being sought was al-shayun, meaning “something,” which was shortened to the symbol for its first “sh” sound. When Spanish scholars translated the Arabic mathematical treatises, they lacked a letter for the “sh” sound and instead chose the “k” sound. They represented this sound by the Greek letter χ, which later became the Latin x.

It’s not unusual for a mathematical expression to come about through convoluted translations—the trigonometric word “sine” started as a Hindu word for a half-chord but, through a series of translations, ended up coming from the Latin word “sinus,” meaning bay. However, there is some evidence that casts doubt upon the theory that using x as an unknown is an artifact of Spanish translation.

The Spanish alphabet includes the letter x, and early Catalonian involved several pronunciations of it depending on context, including a pronunciation akin to the modern sh. Although the sound changed pronunciation over time, there are still vestiges of the sh sound for x in Portuguese, as well as in Mexican Spanish and its use in native place names. By this reasoning, Spanish translators conceivably could have used x without needing to resort first to the Greek χ and then to the Latin x.

Moreover, although the letter x may have been used in mathematics during the Middle Ages sporadically, there is no consistent use of it dating back that far. Western mathematical texts over the next several centuries still used a variety of words, abbreviations and letters to represent the unknown.

For instance, a typical problem in the algebra book “Sumario Compendioso of Juan Diez,” published in Mexico in 1556, uses the word “cosa”—meaning “stuff” or “thing”—to stand in for the unknown.

I think that the most plausible explanation is to credit the influential French scholar René Descartes for the modern use of x. In an appendix to his major work “Discourse” in the 17th century, Descartes introduced a version of analytic geometry—in which algebra is used to solve geometric problems. For unspecified constants he chose the first few letters of the alphabet, and for variables he chose the last letters in reverse order.

Although scholars may never know for sure, some theorize that Descartes may have chosen the letter x to appear often since the printer had a large cache of x’s because of its scarcity in the French language. Whatever his reasons for choosing x, Descartes greatly influenced the development of mathematics, and his mathematical writings were widely circulated.

Xtending beyond algebra

Even if the origins of x in algebra are uncertain, there are some instances in which historians do know why x is used. The X in Xmas as an abbreviation for Christmas definitely does come from the Greek letter χ. The Greek word for Christ is Christos, written χριστοσ and meaning “anointed.” The χ monogram was used as a shorthand for Christ in both Roman Catholic and Eastern Orthodox writings dating back as far as the 16th century.

There are also some contexts in which x was chosen specifically to indicate something unknown or extra, such as when the German physicist Wilhelm Roentgen accidentally discovered X-rays in 1895 while experimenting with cathode rays and glass.

But there are other cases in which scholars can only guess about the origins of x’s role, such as the phrase “X marks the spot.” And there are other contexts—such as Elon Musk’s affinity for the letter—that may just be a matter of personal taste.

For more such insights, log into our website https://international-maths-challenge.com

Credit of the article given to Peter Schumer, The Conversation

Around the world, political violence increased by 27% last year, affecting 1.7 billion people. The numbers come from the Armed Conflict Location & Event Data Project (ACLED), which collects real-time data on conflict events worldwide.

Some armed conflicts occur between states, such as Russia’s invasion of Ukraine. There are, however, many more that take place within the borders of a single state. In Nigeria, violence, particularly from Boko Haram, has escalated in the past few years. In Somalia, populations remain at risk amidst conflict and attacks perpetrated by armed groups, particularly Al-Shabaab.

To address the challenge of understanding how violent events spread, a team at the Complexity Science Hub (CSH) created a mathematical method that transforms raw data on armed conflicts into meaningful clusters by detecting causal links.

“Our main question was: what is a conflict? How can we define it?,” says CSH scientist Niraj Kushwaha, one of the co-authors of the study published in the latest issue of PNAS Nexus. “It was important for us to find a quantitative and bias-free way to see if there were any correlations between different violent events, just by looking at the data.”

“We often tell multiple narratives about a single conflict, which depend on whether we zoom in on it as an example of local tension or zoom out from it and consider it as part of a geopolitical plot; these are not necessarily incompatible,” explains co-author Eddie Lee, a postdoctoral fellow at CSH. “Our technique allows us to titrate between them and fill out a multiscale portrait of conflict.”

In order to investigate the many scales of political violence, the researchers turned to physics and biophysics for inspiration. The approach they developed is inspired by studies of stress propagation in collapsing materials and of neural cascades in the brain.

Kushwaha and Lee used data on violent battles in Africa between 1997 and 2019 from ACLED. In their analysis, they divided the geographic area into a grid of cells and time into sequential slices. The authors predicted when and where new battles would emerge by analysing the presence or absence of battles in each cell over time.

“If there’s a link between two cells, it means a conflict at one location can predict a conflict at another location,” explains Kushwaha. “By using this causal network, we can cluster different conflict events.”

Snow and sandpile avalanches

Observing the dynamics of the clusters, the scientists found that armed clashes spread like avalanches. “In a way evocative of snow or sandpile avalanches, a conflict originates in one place and cascades from there. There is a similar cascading effect in armed conflicts,” explains Kushwaha.

The team also identified a “mesoscale” for political violence —a time scale of a few days to months and a spatial scale of tens to hundreds of kilometers. Violence seems to propagate on these scales, according to Kushwaha and Lee.

Additionally, they found that their conflict statistics matched those from field studies such as in Eastern Nigeria, Somalia, and Sierra Leone. “We connected Fulani militia violence with Boko Haram battles in Nigeria, suggesting that these conflicts are related to one another,” details Kushwaha. The Fulani are an ethnic group living mainly in the Sahel and West Africa.

Policymakers and international agencies could benefit from the approach, according to the authors. The model could help uncover unseen causal links in violent conflicts. Additionally, it could one day help forecast the development of a war at an early stage. “By using this approach, policy decisions could be made more effectively, such as where resources should be allocated,” notes Kushwaha.

For more such insights, log into our website https://international-maths-challenge.com

Credit of the article given to Complexity Science Hub Vienna

Number theory, the study of the properties of positive integers, is perhaps the purest form of mathematics. At first sight, it may seem far too abstract to apply to the natural world. In fact, the influential American number theorist Leonard Dickson wrote, “Thank God that number theory is unsullied by any application.”

And yet, again and again, number theory finds unexpected applications in science and engineering, from leaf angles that (almost) universally follow the Fibonacci sequence, to modern encryption techniques based on factoring prime numbers. Now, researchers have demonstrated an unexpected link between number theory and evolutionary genetics. Their work is published in the Journal of The Royal Society Interface.

Specifically, the team of researchers (from Oxford, Harvard, Cambridge, GUST, MIT, Imperial, and the Alan Turing Institute) have discovered a deep connection between the sums-of-digits function from number theory and a key quantity in genetics, the phenotype mutational robustness. This quality is defined as the average probability that a point mutation does not change a phenotype (a characteristic of an organism).

The discovery may have important implications for evolutionary genetics. Many genetic mutations are neutral, meaning that they can slowly accumulate over time without affecting the viability of the phenotype. These neutral mutations cause genome sequences to change at a steady rate over time. Because this rate is known, scientists can compare the percentage difference in the sequence between two organisms and infer when their latest common ancestor lived.

But the existence of these neutral mutations posed an important question: what fraction of mutations to a sequence are neutral? This property, called the phenotype mutational robustness, defines the average amount of mutations that can occur across all sequences without affecting the phenotype.

Professor Ard Louis from the University of Oxford, who led the study, said, “We have known for some time that many biological systems exhibit remarkably high phenotype robustness, without which evolution would not be possible. But we didn’t know what the absolute maximal robustness possible would be, or if there even was a maximum.”

It is precisely this question that the team has answered. They proved that the maximum robustness is proportional to the logarithm of the fraction of all possible sequences that map to a phenotype, with a correction which is given by the sums of digits function s_k(n), defined as the sum of the digits of a natural number n in base k. For example, for n = 123 in base 10, the digit sum would be s₁₀(123) = 1 + 2 + 3 = 6.

Another surprise was that the maximum robustness also turns out to be related to the famous Tagaki function, a bizarre function that is continuous everywhere, but differentiable nowhere. This fractal function is also called the blancmange curve, because it looks like the French dessert.

First author Dr. Vaibhav Mohanty (Harvard Medical School) added, “What is most surprising is that we found clear evidence in the mapping from sequences to RNA secondary structures that nature in some cases achieves the exact maximum robustness bound. It’s as if biology knows about the fractal sums-of-digits function.”

Professor Ard Louis added, “The beauty of number theory lies not only in the abstract relationships it uncovers between integers, but also in the deep mathematical structures it illuminates in our natural world. We believe that many intriguing new links between number theory and genetics will be found in the future.”

For more such insights, log into our website https://international-maths-challenge.com

Credit of the article given to University of Oxford