Month: May 2022

There are some great uses. Shutterstock

Despite the fact that mathematics is often described as the underpinning science, it is often not given enough credit when scientific discoveries are presented. But the contribution of mathematics and statistics is essential and has transformed entire areas of research – many discoveries would not have been possible without it. In fact, as a mathematician, I have contributed to scientific discoveries and provided solutions to problems that biology was yet to solve.

Seven years ago, I attended a lecture on some biological research that was taking place at Heriot-Watt University. My colleagues had an unsolved problem which related to the movement of bag-like structures called vesicles which move hormones and neurotransmitters such as insulin or serotonin around cells and the body.

Their problem lay in that vesicles were known to follow specific tracks along the cell skeleton which lead to special molecules which then caused the vesicle to release its contents into the cell. However, when the biologists themselves tried to find these tracks, they were not in the expected places.

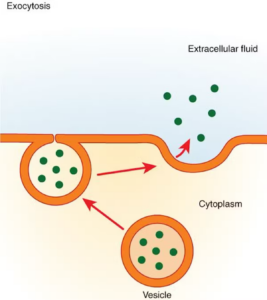
A bag that carries hormones to their location. OpenStax, CC BY

It is important to understand how vesicles behave, or in fact misbehave, as they have been linked to conditions such diabetes and neurological disorders. The biologists were struggling to find a way to understand the vesicles – but I had a solution in my mathematical toolkit.

Maths can beat biology

After two years of collaboration I told my colleagues: “my model and computer experiments are better than your microscope!”

What I meant by this rather confident statement was that by using mathematics to model how molecules move in a cell we could predict and run multiple experiments on a computer at a smaller scale and faster rate than a microscope. It could allow us to uncover things that the biologist’s resources could not, and might even point us in the direction of target molecules for future treatments of diabetes and neurological disorders.

The mathematical model allowed us to recognise that the movement of vesicles requires energy – and the maths models it through an energy landscape. It imagined a vesicle to be like a cyclist riding a bicycle – the landscape may have easy level sections but also hills that require more energy input to get over them, and so we wanted to test whether they actually avoided these hills.

After seven years of working in partnership with the biologists, my colleagues and I proved our hypothesis was correct. Vesicles do follow lower energy “valleys” in the landscape, avoiding molecules which create the high energy hills in the energy landscape – taking the easiest path. The overall result is just the same as the biologists had found – the vesicles end up in the same end location and they reuse similar routes over and over again. But the difference lies in the way in which they do it, and it was not by following the cell skeleton as biologists had first believed – they take an easier route. It really shows the power of maths and how it can change the way we see things.

Mathematical models allow you to capture many gigabytes of raw data in a compact form in a way that is impossible for a biologist with a microscope. You can make modifications to the model easily and show how vesicle behaviour may change during disease, when they are disrupted or mutated. It could then reveal which molecules to target in future treatment studies – and lay the groundwork for larger and more thorough modelling of complex biological processes.

A modelled energy landscape. Shutterstock

The integration of cutting-edge microscopy with cell biology and mathematical modelling could be applied to many other problems in bio-medicine and will accelerate discovery in the years to come. The movement of molecules and other cell components is just one example of where the power of mathematics is unrivalled, but it is by no means its limit.

Useful is an understatement

Maths is often criticised by the public for lacking in “real-world” applications, but it is being applied to many real-world problems all the time. Groundwater contamination, financial and economic forecasting, plume heights in volcanic eruptions, the modelling of biological processes and drug delivery are just a few places where maths is making a huge difference.

I’m proud to say that I co-authored a paper with my biology colleagues, and I hope to see more mathematicians coming to the fore for science research in the future. Mathematics plays a central role in so many of the world’s scientific breakthroughs and deserves a headline role in more academic publications. Power to the mathematician – they’re behind more discoveries than you think.

For more insights like this, visit our website at www.international-maths-challenge.com.
Credit of the article given to Gabriel Lord

A small dot on an old piece of birch bark marks one of the biggest events in the history of mathematics. The bark is actually part of an ancient Indian mathematical document known as the Bakhshali manuscript. And the dot is the first known recorded use of the number zero. What’s more, researchers from the University of Oxford recently discovered the document is 500 years older than was previously estimated, dating to the third or fourth century – a breakthrough discovery.

Today, it’s difficult to imagine how you could have mathematics without zero. In a positional number system, such as the decimal system we use now, the location of a digit is really important. Indeed, the real difference between 100 and 1,000,000 is where the digit 1 is located, with the symbol 0 serving as a punctuation mark.

Yet for thousands of years we did without it. The Sumerians of 5,000BC employed a positional system but without a 0. In some rudimentary form, a symbol or a space was used to distinguish between, for example, 204 and 20000004. But that symbol was never used at the end of a number, so the difference between 5 and 500 had to be determined by context.

What’s more, 0 at the end of a number makes multiplying and dividing by 10 easy, as it does with adding numbers like 9 and 1 together. The invention of zero immensely simplified computations, freeing mathematicians to develop vital mathematical disciplines such as algebra and calculus, and eventually the basis for computers.

Zero’s late arrival was partly a reflection of the negative views some cultures held for the concept of nothing. Western philosophy is plagued with grave misconceptions about nothingness and the mystical powers of language. The fifth century BC Greek thinker Parmenides proclaimed that nothing cannot exist, since to speak of something is to speak of something that exists. This Parmenidean approach kept prominent historical figures busy for a long while.

After the advent of Christianity, religious leaders in Europe argued that since God is in everything that exists, anything that represents nothing must be satanic. In an attempt to save humanity from the devil, they promptly banished zero from existence, though merchants continued secretly to use it.

By contrast, in Buddhism the concept of nothingness is not only devoid of any demonic possessions but is actually a central idea worthy of much study en route to nirvana. With such a mindset, having a mathematical representation for nothing was, well, nothing to fret over. In fact, the English word “zero” is originally derived from the Hindi “sunyata”, which means nothingness and is a central concept in Buddhism.

The Bakhshali manuscript. Bodleian Libraries

So after zero finally emerged in ancient India, it took almost 1,000 years to set root in Europe, much longer than in China or the Middle East. In 1200 AD, the Italian mathematician Fibonacci, who brought the decimal system to Europe, wrote that:

The method of the Indians surpasses any known method to compute. It’s a marvellous method. They do their computations using nine figures and the symbol zero.

This superior method of computation, clearly reminiscent of our modern one, freed mathematicians from tediously simple calculations, and enabled them to tackle more complicated problems and study the general properties of numbers. For example, it led to the work of the seventh century Indian mathematician and astronomer Brahmagupta, considered to be the beginning of modern algebra.

Algorithms and calculus

The Indian method is so powerful because it means you can draw up simple rules for doing calculations. Just imagine trying to explain long addition without a symbol for zero. There would be too many exceptions to any rule. The ninth century Persian mathematician Al-Khwarizmi was the first to meticulously note and exploit these arithmetic instructions, which would eventually make the abacus obsolete.

Such mechanical sets of instructions illustrated that portions of mathematics could be automated. And this would eventually lead to the development of modern computers. In fact, the word “algorithm” to describe a set of simple instructions is derived from the name “Al-Khwarizmi”.

The invention of zero also created a new, more accurate way to describe fractions. Adding zeros at the end of a number increases its magnitude, with the help of a decimal point, adding zeros at the beginning decreases its magnitude. Placing infinitely many digits to the right of the decimal point corresponds to infinite precision. That kind of precision was exactly what 17th century thinkers Isaac Newton and Gottfried Leibniz needed to develop calculus, the study of continuous change.

And so algebra, algorithms, and calculus, three pillars of modern mathematics, are all the result of a notation for nothing. Mathematics is a science of invisible entities that we can only understand by writing them down. India, by adding zero to the positional number system, unleashed the true power of numbers, advancing mathematics from infancy to adolescence, and from rudimentary toward its current sophistication.

For more insights like this, visit our website at www.international-maths-challenge.com.
Credit of the article given to Ittay Weiss

Credit: Monty Rakusen Getty Images

Scientists have created a “map” of odor molecules, which could ultimately be used to predict new scent combinations 

The human nose finds it simple to distinguish the aroma of fresh coffee from the stink of rotten eggs, but the underlying biochemistry is complicated. Researchers have now created an olfactory “map”—a geometric model of how molecules combine to produce various scents. This map could inspire a way to predict how people might perceive certain odor combinations and help to drive the development of new fragrances, scientists say.

Researchers have been trying for years to tame the elaborate landscape of odor molecules. Neuroscientists want to better understand how we process scents; perfume and food manufacturers want better ways to synthesize familiar aromas for their products. The new approach may appeal to both camps.

One earlier strategy for mapping the olfactory system involves grouping odor molecules that have similar molecular structures and using those similarities to predict the scents of novel combinations. But that avenue often leads to a dead end. “It’s not necessary that chemicals with the same chemical structures will be perceived similarly,” says Tatyana Sharpee, a neurobiologist at the Salk Institute for Biological Studies in La Jolla, Calif., and lead author of the study, which appeared in August in Science Advances.

Sharpee and her colleagues analyzed odor molecules found in four familiar and unmistakable scents: strawberries, tomatoes, blueberries and mouse urine. The researchers calculated how often and in what concentrations certain molecules turned up together in these scents. They then created a mathematical model in which molecules that occurred together frequently were represented as closer in space and molecules that rarely did so were farther apart. The result was a “saddle”-shaped surface—a hallmark of a field called hyperbolic geometry, which obeys different rules from the geometry most people learn in school.

The researchers envision an algorithm, trained on this hyperbolic geometry model, that can predict the scents of new odor combinations—or even help to synthesize them. One of Sharpee’s collaborators, behavioral neuroscientist Brian Smith of Arizona State University, wants to use this method to create olfactory environments in places devoid of natural scents.

Such a tool would be useful to scientists and odor manufacturers alike, says olfactory neuroscientist Joel Mainland of the Monell Chemical Senses Center in Philadelphia, who was not involved in the study. The ultimate goal is to know enough about how odors work to replicate natural smells without the natural sources, Mainland says: “We want to identify a strawberry flavor without worrying about replicating the ingredients that are in a strawberry.”

For more insights like this, visit our website at www.international-maths-challenge.com.

Credit of the article given to Stephen Ornes