Month: September 2021

March 21 marks the 250th birthday of one of the most influential mathematicians in history. He accompanied Napoleon on his expedition to Egypt, revolutionized science’s understanding of heat transfer, developed the mathematical tools used today to create CT and MRI scan images, and discovered the greenhouse effect.

His name was Joseph Fourier. He wrote of mathematics: “There cannot be a language more universal and more simple, more free from errors and obscurities … Mathematical analysis is as extensive as nature itself, and it defines all perceptible relations.” Fourier’s work continues to shape life today, especially for people like ourselves working in fields such as mathematics and radiology.

Fourier’s life

Mathematician and physicist Joseph Fourier. Wikimedia Commons

As a troubled orphan in France, Fourier was transformed by his first encounter with mathematics. Thanks to a local bishop who recognized his talent, Fourier received an education through Benedictine monks. As a college student, he so loved math that he collected discarded candle stumps so he could continue his studies after others had gone to bed.

As a young man, Fourier was soon swept up by the French Revolution. However, he became disenchanted by its excessive brutality, and his protests landed him in prison for part of 1794. After his release, he was appointed to the faculty of an engineering school. There he proved his genius by substituting for ill colleagues, teaching subjects ranging from physics to classics.

Traveling with Napoleon to Egypt in 1798, Fourier was appointed secretary of the Egyptian Institute, which Napoleon modeled on the Institute of France. When the British fleet stranded the French forces, he organized the manufacture of weapons and munitions to permit the French to continue fighting. Fourier returned to France after the British navy forced the French to surrender. Even in the midst of such difficult circumstances, he managed to publish a number of mathematical papers.

Heat transfer

One of the most important fruits of Fourier’s studies concerns heat.

Fourier’s law states that heat transfers through a material at a rate proportional to both the difference in temperature between different areas and to the area across which the transfer takes place. For example, people who are overheated can cool off quickly by getting to a cool place and exposing as much of their body to it as possible.

Fourier’s work enables scientists to predict the future distribution of heat. Heat is transferred through different materials at different rates. For example, brass has a high thermal conductivity. Air is poorly conductive, which is why it’s frequently used in insulation.

Remarkably, Fourier’s equation applies widely to matter, whether in the form of solid, liquid or gas. It powerfully shaped scientists’ understanding of both electricity and the process of diffusion. It also transformed scientists’ understanding of flow in nature generally – from water’s passage through porous rocks to the movement of blood through capillaries.

Fourier transform and CT

Today, when helping to care for patients, radiologists rely on another mathematical discovery of Fourier’s, now referred to as the “Fourier transform.”

In CT scans, doctors send X-ray beams through a patient from multiple different directions. Some X-rays emerge from the other side, where they can be measured, while others are blocked by structures within the body.

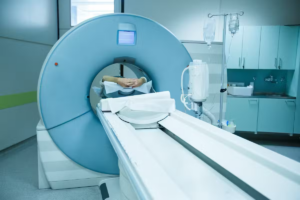
Modern medical imaging machines rely on Fourier’s transform. zlikovec/shutterstock.com

With many such measurements taken at many different angles, it becomes possible to determine the degree to which each tiny block of tissue blocked the beam. For example, bone blocks most of the X-rays, while the lungs block very little. Through a complex series of computations, it’s possible to reconstruct the measurements into two-dimensional images of a patient’s internal anatomy.

Thanks to Fourier and today’s powerful computers, doctors can create almost instantaneous images of the brain, the pulmonary arteries, the appendix and other parts of the body. This in turn makes it possible to confirm or rule out the presence of issues such as blood clots in the pulmonary arteries or inflammation of the appendix. It’s difficult to imagine practicing medicine today without such CT images.

Greenhouse effect

Fourier is generally regarded as the first scientist to notice what we today call the greenhouse effect.

His interest was piqued when he observed that a planet as far away from the sun as Earth should be considerably cooler. He hypothesized that something about the Earth – in particular, its atmosphere – must enable it to trap solar radiation that would otherwise simply radiate back out into space.

Fourier created a model of the Earth involving a box with a glass cover. Over time, the temperature in the box rose above that of the surrounding air, suggesting that the glass continually trapped heat. Because his model resembled a greenhouse in some respects, this phenomenon came to be called the “greenhouse effect.”

Later, scientist John Tyndall discovered that carbon dioxide can play the role of heat trapper.

Life on earth as we know it would not be possible without the greenhouse effect. However, today scientists tend to be more concerned about an excess of greenhouse gases. Mathematical models suggest that as carbon dioxide accumulates, heat may be trapped more quickly, resulting in elevated global average temperatures, melting polar ice caps and rising sea levels.

Fourier’s impact

Fourier received many honors during his lifetime, including election to the French Academy of Science.

Some believed, perhaps speciously, that Fourier’s attraction to heat may have hastened his death. He was known to climb into saunas in multiple layers of clothes, and his acquaintances claimed that he kept his rooms hotter than Hades. At any rate, in May 1830, he died of an aneurysm at the age of 63.

Today, Fourier’s name is inscribed on the Eiffel Tower. But more importantly, it is immortalized in Fourier’s law and the Fourier transform, enduring emblems of his belief that mathematics holds the key to the universe.

For more insights like this, visit our website at www.international-maths-challenge.com.
Credit of the article given to Richard Gunderman, David Gunderman

Credit: ©iStock.com

Last digits of nearby primes have “anti-sameness” bias

Two mathematicians have found a strange pattern in prime numbers—showing that the numbers are not distributed as randomly as theorists often assume.

“Every single person we’ve told this ends up writing their own computer program to check it for themselves,” says Kannan Soundararajan, a mathematician at Stanford University in California, who reported the discovery with his colleague Robert Lemke Oliver in a paper submitted to the arXiv preprint server on March 11. “It is really a surprise,” he says.

Prime numbers near to each other tend to avoid repeating their last digits, the mathematicians say: that is, a prime that ends in 1 is less likely to be followed by another ending in 1 than one might expect from a random sequence. “As soon as I saw the numbers, I could see it was true,” says mathematician James Maynard of the University of Oxford, UK. “It’s a really nice result.”

Although prime numbers are used in a number of applications, such as cryptography, this ‘anti-sameness’ bias has no practical use or even any wider implication for number theory, as far as Soundararajan and Lemke Oliver know. But, for mathematicians, it’s both strange and fascinating.

Not so random

A clear rule determines exactly what makes a prime: it’s a whole number that can’t be exactly divided by anything except 1 and itself. But there’s no discernable pattern in the occurrence of the primes. Beyond the obvious—after the numbers 2 and 5, primes can’t be even or end in 5—there seems to be little structure that can help to predict where the next prime will occur.

As a result, number theorists find it useful to treat the primes as a ‘pseudorandom’ sequence, as if it were created by a random-number generator.

But if the sequence were truly random, then a prime with 1 as its last digit should be followed by another prime ending in 1 one-quarter of the time. That’s because after the number 5, there are only four possibilities—1, 3, 7 and 9—for prime last digits. And these are, on average, equally represented among all primes, according to a theorem proved around the end of the nineteenth century, one of the results that underpin much of our understanding of the distribution of prime numbers. (Another is the prime number theorem, which quantifies how much rarer the primes become as numbers get larger.)

Instead, Lemke Oliver and Soundararajan saw that in the first billion primes, a 1 is followed by a 1 about 18% of the time, by a 3 or a 7 each 30% of the time, and by a 9 22% of the time. They found similar results when they started with primes that ended in 3, 7 or 9: variation, but with repeated last digits the least common. The bias persists but slowly decreases as numbers get larger.

The k-tuple conjecture

The mathematicians were able to show that the pattern they saw holds true for all primes, if a widely accepted but unproven statement called the Hardy–Littlewood k-tuple conjecture is correct. This describes the distributions of pairs, triples and larger prime clusters more precisely than the basic assumption that the primes are evenly distributed.

The idea behind it is that there are some configurations of primes that can’t occur, and that this makes other clusters more likely. For example, consecutive numbers cannot both be prime—one of them is always an even number. So if the number n is prime, it is slightly more likely that n + 2 will be prime than random chance would suggest. The k-tuple conjecture quantifies this observation in a general statement that applies to all kinds of prime clusters. And by playing with the conjecture, the researchers show how it implies that repeated final digits are rarer than chance would suggest.

At first glance, it would seem that this is because gaps between primes of multiples of 10 (20, 30, 100 and so on) multiples of 10 are disfavoured. But the finding gets much more general—and even more peculiar. A prime’s last digit is its remainder when it is divided by 10. But the mathematicians found that the anti-sameness bias holds for any divisor. Take 6, for example. All primes have a remainder of 1 or 5 when divided by 6 (otherwise, they would be divisible by 2 or 3) and the two remainders are on average equally represented among all primes. But the researchers found that a prime that has a remainder of 1 when divided by 6 is more likely to be followed by one that has a remainder of 5 than by another that has a remainder of 1. From a 6-centric point of view, then, gaps of multiples of 6 seem to be disfavoured.

Paradoxically, checking every possible divisor makes it appear that almost all gaps are disfavoured, suggesting that a subtler explanation than a simple accounting of favoured and disfavoured gaps must be at work. “It’s a completely weird thing,” says Soundararajan.

Mystifying phenomenon

The researchers have checked primes up to a few trillion, but they think that they have to invoke the k-tuple conjecture to show that the pattern persists. “I have no idea how you would possibly formulate the right conjecture without assuming it,” says Lemke Oliver.

Without assuming unproven statements such as the k-tuple conjecture and the much-studied Riemann hypothesis, mathematicians’ understanding of the distribution of primes dries up. “What we know is embarrassingly little,” says Lemke Oliver. For example, without assuming the k-tuple conjecture, mathematicians have proved that the last-digit pairs 1–1, 3–3, 7–7 and 9–9 occur infinitely often, but they cannot prove that the other pairs do. “Perversely, given our work, the other pairs should be more common,” says Lemke Oliver.

He and Soundararajan feel that they have a long way to go before they understand the phenomenon on a deep level. Each has a pet theory, but none of them is really satisfying. “It still mystifies us,” says Soundararajan.

For more insights like this, visit our website at www.international-maths-challenge.com.

Credit of the article given to Evelyn Lamb & Nature magazine