Month: January 2021

The humble honeybee can use symbols to perform basic maths including addition and subtraction, shows new research published today in the journal Science Advances.

Despite having a brain containing less than one million neurons, the honeybee has recently shown it can manage complex problems – like understanding the concept of zero.

Honeybees are a high value model for exploring questions about neuroscience. In our latest study we decided to test if they could learn to perform simple arithmetical operations such as addition and subtraction.

Addition and subtraction operations

As children, we learn that a plus symbol (+) means we have to add two or more quantities, while a minus symbol (-) means we have to subtract quantities from each other.

To solve these problems, we need both long-term and short-term memory. We use working (short-term) memory to manage the numerical values while performing the operation, and we store the rules for adding or subtracting in long-term memory.

Although the ability to perform arithmetic like adding and subtracting is not simple, it is vital in human societies. The Egyptians and Babylonians show evidence of using arithmetic around 2000BCE, which would have been useful – for example – to count live stock and calculate new numbers when cattle were sold off.

This scene depicts a cattle count (copied by the Egyptologist Lepsius). In the middle register we see 835 horned cattle on the left, right behind them are some 220 animals and on the right 2,235 goats. In the bottom register we see 760 donkeys on the left and 974 goats on the right. Wikimedia commons, CC BY

But does the development of arithmetical thinking require a large primate brain, or do other animals face similar problems that enable them to process arithmetic operations? We explored this using the honeybee.

How to train a bee

Honeybees are central place foragers – which means that a forager bee will return to a place if the location provides a good source of food.

We provide bees with a high concentration of sugar water during experiments, so individual bees (all female) continue to return to the experiment to collect nutrition for the hive.

In our setup, when a bee chooses a correct number (see below) she receives a reward of sugar water. If she makes an incorrect choice, she will receive a bitter tasting quinine solution.

We use this method to teach individual bees to learn the task of addition or subtraction over four to seven hours. Each time the bee became full she returned to the hive, then came back to the experiment to continue learning.

Addition and subtraction in bees

Honeybees were individually trained to visit a Y-maze shaped apparatus.

The bee would fly into the entrance of the Y-maze and view an array of elements consisting of between one to five shapes. The shapes (for example: square shapes, but many shape options were employed in actual experiments) would be one of two colours. Blue meant the bee had to perform an addition operation (+ 1). If the shapes were yellow, the bee would have to perform a subtraction operation (- 1).

For the task of either plus or minus one, one side would contain an incorrect answer and the other side would contain the correct answer. The side of stimuli was changed randomly throughout the experiment, so that the bee would not learn to only visit one side of the Y-maze.

After viewing the initial number, each bee would fly through a hole into a decision chamber where it could either choose to fly to the left or right side of the Y-maze depending on operation to which she had been trained for.

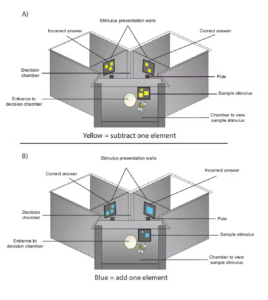
The Y-maze apparatus used for training honeybees. Scarlett Howard

At the beginning of the experiment, bees made random choices until they could work out how to solve the problem. Eventually, over 100 learning trials, bees learnt that blue meant +1 while yellow meant -1. Bees could then apply the rules to new numbers.

During testing with a novel number, bees were correct in addition and subtraction of one element 64-72% of the time. The bee’s performance on tests was significantly different than what we would expect if bees were choosing randomly, called chance level performance (50% correct/incorrect)

Thus, our “bee school” within the Y-maze allowed the bees to learn how to use arithmetic operators to add or subtract.

Why is this a complex question for bees?

Numerical operations such as addition and subtraction are complex questions because they require two levels of processing. The first level requires a bee to comprehend the value of numerical attributes. The second level requires the bee to mentally manipulate numerical attributes in working memory.

In addition to these two processes, bees also had to perform the arithmetic operations in working memory – the number “one” to be added or subtracted was not visually present. Rather, the idea of plus one or minus “one” was an abstract concept which bees had to resolve over the course of the training.

Showing that a bee can combine simple arithmetic and symbolic learning has identified numerous areas of research to expand into, such as whether other animals can add and subtract.

For more insights like this, visit our website at www.international-maths-challenge.com.
Credit of the article given to Scarlett Howard, Adrian Dyer, Jair Garcia

Credit: If you know your height, can you predict how big your arm span is? What about the length of your femur? Or the circumference of your head? Try this simple activity and find out how you knowing these simple ratios can even make you a better artist!  George Retseck

A project that measures up

Introduction
Our bodies are amazing! They are full of mysteries and surprising facts such as this one: Did you know that you are about a centimeter taller in the morning, when you have just woken up after hours of lying down, than you are in the evening? You might never have noticed it. These interesting facts only reveal themselves when you look closely, measure and compare. That is what this activity is about: recording, comparing and discovering how the human body measures up!

Background
Did you know that human bodies come in all sizes and forms? When you start measuring them, however, you will find our bodies show surprising similarities—and even more surprisingly, we can express these with mathematical concepts.

For one thing, our bodies are quite symmetrical. When you draw a vertical line down the center of a body, the left and right sides are almost mirror images of each other. Human bodies also show interesting ratios. Ratios compare two quantities, like the size of one part of the body to the size of another part, or to the size of the whole. An example of a human body ratio is a person’s arm span—the distance from the middle fingertip of the left hand to that of the right hand when stretching out both arms horizontally—to their height. This ratio is approximately a one to one ratio, meaning that a person’s arm span is about equal to their height. There are many more human body ratios; some are independent of age, and others change as we grow from a baby to an adult.

Wondering who would be interested in these ratios? Artists are avid users of human body ratios, because it helps them draw realistic-looking figures. They are also used in the medical world; a sizable deviation from a human body ratio can indicate a body that does not develop according to expectations. In this science activity we will examine some human body ratios and, if you like, we can explore how they can help you draw more realistic-looking figures.

Materials

• Yarn
• Scissors
• A hardcover book
• A helper
• Pen and paper (optional)
• Measuring tape (optional)

Preparation

• To compare the length of different parts of your body with your height, we will first create a string the length of your height. Take off your shoes. The easiest way is to lie on the ground with your heels pressing against a wall. Look straight up and have your helper place a hardcover book flat against the top of your head, resting on the ground. Get out from under the book and, together, span the yarn across the floor from the wall to the book, cutting the yarn just where it reaches it. Now you have a piece of yarn that is as long as you are tall. (If lying on the ground is not possible, you can also stand flat on the floor against the wall and have the book rest on top of your head and against the wall.)

Procedure

• First, we examine your arm span to height ratio. Your arm span is the distance between the middle fingertips on each hand when you stretch your arms out as far as they can reach. How do you think your height compares with your arm span? Would it be similar, way longer or way shorter?
• Now stretch your arms out as far as they can reach. Your arms will be parallel to the ground. Hold one end of the piece of yarn you just cut off with the fingertips of your left hand. Let your helper span the yarn toward the tip of your right hand’s middle finger. Is piece long enough, way longer or way too short? What does this tell you about how your arm span compares to your height?
• For most people, their arm span is about equal to their height. Mathematicians say the arm span to height ratio is one to one: your arm span goes once into your height.
• Now let’s explore another ratio: the length of your femur bone to your height. The femur bone is the only bone in your thigh. To measure its length, sit down and span a new piece of yarn over your thigh from the hip joint to the edge of your knee and cut the yarn there.
• Make an estimate. How many times would this piece of yarn go into the piece that is as long as you are tall? Can you find a way to test your estimate?
• There are several ways to compare the length of the two pieces of yarn: You might cut several pieces of the length of your shorter string, lay them end to end next to your longer piece, and count how many you need. Another way is to fold the longer string into equal parts so the length of the folded string equals the length of the shorter string. The number of folds needed is exactly the number of times your shorter string goes into your longer string.
• Did you see that the length of your femur bone goes about four times into your height? You can also say that if you divide your height in four equal pieces, you have the length of your femur bone, or the length of your femur bone is one fourth of your height. Mathematicians call this a one to four ratio.
• Now let’s move on to a ratio that might help you make more realistic drawings: the head to body ratio. How many times would the length of your head fit into your height? Maybe four, six or eight times? To test six times, fold the yarn with length equaling your height into six equal pieces. Have your helper place a book flat on your head and hang the folded string from the side of the book. If the other end of the string is about level with your chin, your height would be about six times the length of your head, or your head to body ratio would be one to six. Which number of folds fits best for you?
• There are many more bodily ratios you can explore: the circumference of your head compared with your height, or the length ratios of your forearm and foot or thumb and hand. Use pieces of yarn to measure, compare and detect these and/or your other bodily ratios.
• Extra: You have explored some ratios in your body and might wonder if these hold for other people as well. Do you think they hold for most people of your age? What about adults or babies? Do you think these ratios hold for them or would some be different? Make a hypothesis, find some volunteers, measure and compare. Was your hypothesis correct?
• Extra: This activity uses pieces of yarn to compare lengths. You can also measure your height, arm span, femur bone, etcetera with measuring tape, round the values and write the ratios as fractions. Can you find a way to simplify these fractions?
• Extra: Draw some stick figures on a sheet of paper. Can you apply some of the bodily ratios you explored (like the arm span to height or the head to body ratio) to the figures?Which ones look most realistic to you?
• Extra: Ratios are all around us. Can you find other places where ratios play an important role? To get you started, think about a recipe and the ratio of the quantity of one ingredient to another. For avid bikers, can you find the ratios that correspond to the different gears on a bike?

Observations and results
You probably found your arm span to height ratio approximately to be one to one whereas the femur to height was approximately one to four. This is expected because on average and over a large age range the human body has an arm span that is roughly equal to its height and a femur bone roughly a quarter of its height.

The head to body ratio is a little more complex as it changes from a ratio of about one to four for a small child to about one to eight for an adult. A five-year-old is likely to have a head to body ratio of about one to six.

It is good to remember these ratios are averages over a large group of people. Individual variations occur; some might even be used to one’s advantage—for example, having exceptionally long arms can be advantageous when playing basketball.

For more insights like this, visit our website at www.international-maths-challenge.com.

Credit of the article given to Science Buddies & Sabine De Brabandere