Have you ever taken a walk through the rain on a warm spring day and seen that perfect puddle? You know, the one where the raindrops seem to touch down at just the right pace, causing a dance of vanishing circles?
Even before I entered the field of fluid flow research nearly 15 years ago, I was fascinated by the waves that appear after a raindrop hits a puddle.
As I became focused on the study of unstable waves in liquid sheets – geared toward mitigating undesirable waves in industrial coating and atomization processes – my fascination with puddle waves turned into an obsession. What is going on? Where does the pattern come from? Why does the impact of rain in a puddle look different than when rain falls elsewhere, like in a lake or the ocean?
It turns out that it all has to do with something called dispersion.
In the context of water waves, dispersion is the ability of waves of different wavelengths to each move at their own individual speeds. Looking down on a puddle, we see a collection of such waves moving together as one ripple in the water.
When a raindrop touches down, imagine it as a “ding” to the water surface. This ding can be idealized as a packet of waves of all different sizes. After the raindrop falls, the packet’s waves are ready to begin their new life in the puddle.
However, whether we see those waves as ripples depends on the body of water that the raindrop lands on. The number and spacing of rings that you see depends on the height of the puddle. This has been verified in some very cool ripple tank experiments, where a drop of the same velocity falls into a container with water at different depths.
Shallow puddles enable ripples, because they are much thinner than they are wide. The balance between the surface force – between the water puddle and the air above it – and the gravitational force tips in favor of surface force. This is key, since the surface force depends on the curvature of the water surface, whereas the gravitational force does not.
An initially still shallow puddle becomes curved at the surface after the raindrop hits. The surface force is different for long waves than for short ones, causing waves of different sizes to separate into ripples. For shallow puddles, the long waves move slowly away from the point of impact, while the short waves move fast, and the really short waves move really fast, becoming tightly packed at the perimeter. This creates the enchanting pattern that we see.
Raindrops may react differently in other situations. Imagine that rain is hitting a lake or ocean – or those deep pothole puddles that require galoshes. Here, the raindrop hits the water, but the force due to gravity becomes more important. It moves waves of all sizes at the same speed which may overpower the rippling effect due to the surface force.
The combination of teaching undergraduate partial differential equations while simultaneously continuing to research liquid sheets led to what I’ve been calling the “puddle equation.” When solved, the equation creates an animated simulation of what happens after a raindrop hits a puddle. It’s a simplified version of an equation in one of our group’s more recent research endeavors, but it’s also consistent with the classical description of ripples.
I use this approximate description of puddle waves as one way to get students excited about math by relating it to the world around them.
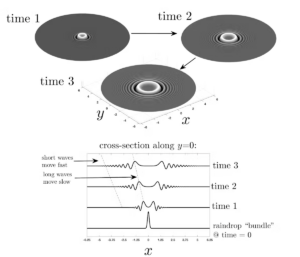
A model of waves in a dispersive puddle, after a raindrop hits. The top three figures show what happens after a drop hits the puddle, with arrows indicating the passage of time. The bottom figure shows the cross-sectional view through the puddle, highlighting that the initial wave bundle caused by the raindrop splits into waves of different sizes. Large waves in the center move more slowly than small waves at the perimeter. Nate Barlow
The study of surface-force-driven waves is important for applications such as coating processes involved in making batteries and solar cells.
Such waves also appear as a result of the leg stroke of a water strider insect, but research has found that the water strider isn’t specifically looking to make those waves to enable travel.
The beauty of puddle waves is no small thing by itself. By connecting nature with its primal language – mathematics – we gain access to its control panel, allowing us to observe every little detail, uncovering all the secrets.
For more insights like this, visit our website at www.international-maths-challenge.com.
Credit of the article given to Nate Barlow


