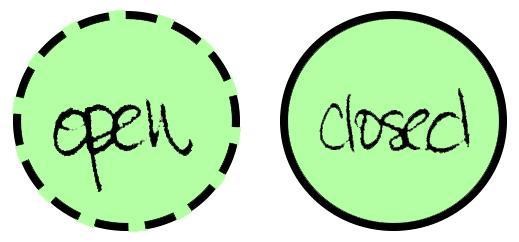
When you hear the word closure, what do you think of? I think of wholeness – you know, tying loose ends, wrapping things up, filling in the missing parts. This same idea is behind the mathematician’s notion of closure, as in the phrase “taking the closure” of a set. Intuitively this just means adding in any missing pieces so that the result is complete, whole. For instance, the circle on the left is open because it’s missing its boundary. But when we take its closure and include the boundary, we say the circle is closed.
As another example, consider the set of all real numbers strictly between 0 and 1, i.e. the open interval (0,1). Notice that we can get arbitrarily close to 0, but we can’t quite reach it. In some sense, we feel that 0 might as well be included in set, right? I mean, come on, 0.0000000000000000000000000000000000000001 is basically 0, right? So by not considering 0 as an element in our set, we feel like something’s missing. The same goes for 1.
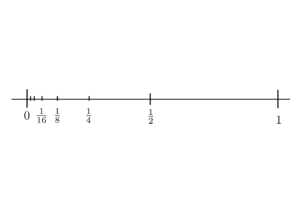
We say an element is a limit point of a given set if that element is “close” to the set,* and we say the set’s closure is the set together with its limit points. (So 0 and 1 are both limit points of (0,1) and its closure is [0,1].) It turns out the word closure is also used in algebra, specifically the algebraic closure of a field, but there it has a completely different definition which has to do with roots of polynomials, called algebraic elements. Now why would mathematicians use the same word to describe two seemingly different things? The purpose of today’s post is to make the observation that they’re not so different after all! This may be somewhat obvious, but it wasn’t until after a recent conversation with a friend that I saw the connection:
algebraic elements of a field
are like
limit points of a sequence!
(Note: I’m not claiming any theorems here, this is just a student’s simple observation.)
For more such insights, log into www.international-maths-challenge.com.
*Credit for article given to Tai-Danae Bradley*