Not content with shapes in two or three dimensions, mathematicians like to explore objects in any number of spatial dimensions. Now they have discovered shapes of constant width in any dimension, which roll like a wheel despite not being round.
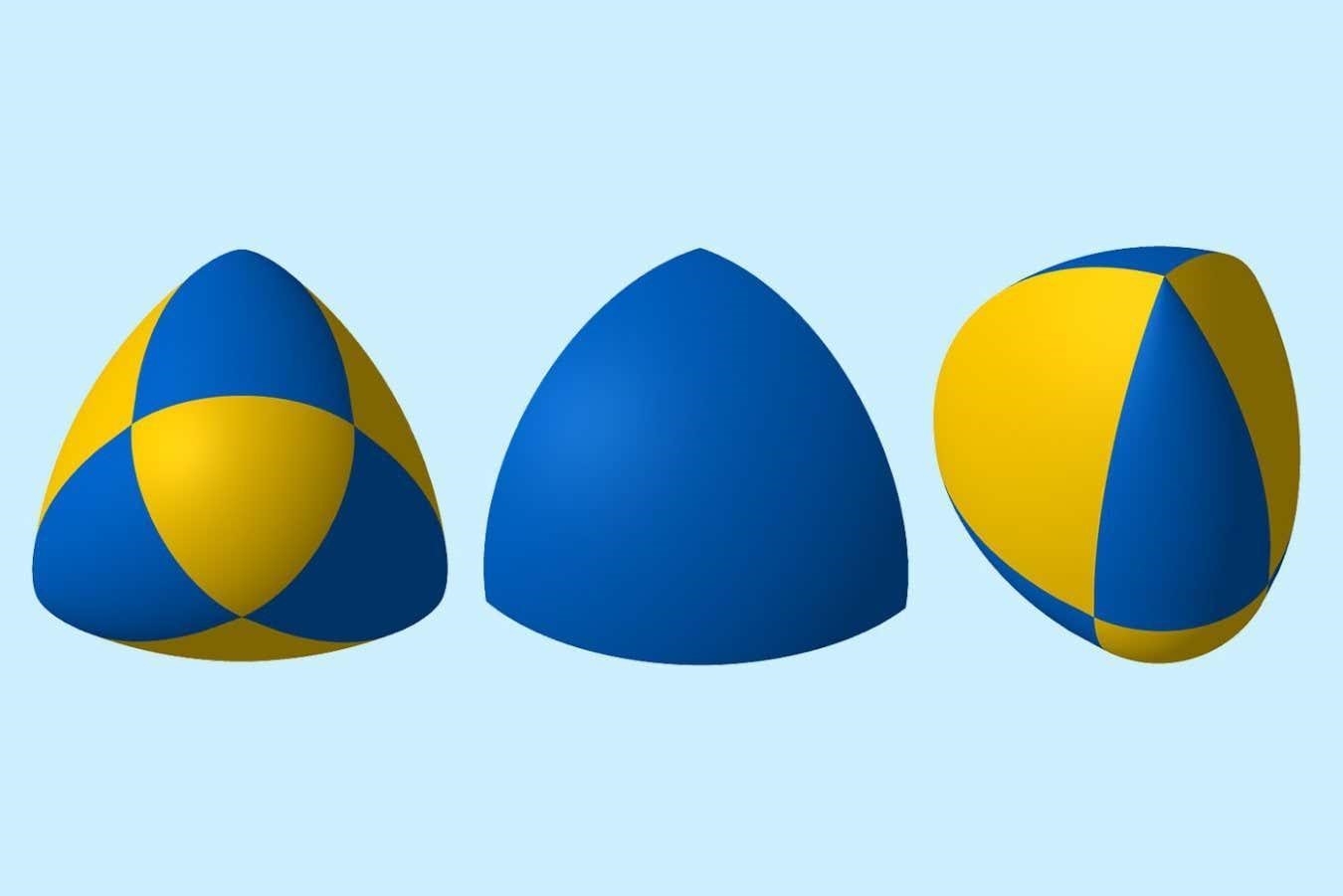
A 3D shape of constant width as seen from three different angles. The middle view resembles a 2D Reuleaux triangle
Mathematicians have reinvented the wheel with the discovery of shapes that can roll smoothly when sandwiched between two surfaces, even in four, five or any higher number of spatial dimensions. The finding answers a question that researchers have been puzzling over for decades.
Such objects are known as shapes of constant width, and the most familiar in two and three dimensions are the circle and the sphere. These aren’t the only such shapes, however. One example is the Reuleaux triangle, which is a triangle with curved edges, while people in the UK are used to handling equilateral curve heptagons, otherwise known as the shape of the 20 and 50 pence coins. In this case, being of constant width allows them to roll inside coin-operated machines and be recognised regardless of their orientation.
Crucially, all of these shapes have a smaller area or volume than a circle or sphere of the equivalent width – but, until now, it wasn’t known if the same could be true in higher dimensions. The question was first posed in 1988 by mathematician Oded Schramm, who asked whether constant-width objects smaller than a higher-dimensional sphere might exist.
While shapes with more than three dimensions are impossible to visualise, mathematicians can define them by extending 2D and 3D shapes in logical ways. For example, just as a circle or a sphere is the set of points that sits at a constant distance from a central point, the same is true in higher dimensions. “Sometimes the most fascinating phenomena are discovered when you look at higher and higher dimensions,” says Gil Kalai at the Hebrew University of Jerusalem in Israel.
Now, Andrii Arman at the University of Manitoba in Canada and his colleagues have answered Schramm’s question and found a set of constant-width shapes, in any dimension, that are indeed smaller than an equivalent dimensional sphere.
Arman and his colleagues had been working on the problem for several years in weekly meetings, trying to come up with a way to construct these shapes before they struck upon a solution. “You could say we exhausted this problem until it gave up,” he says.
The first part of the proof involves considering a sphere with n dimensions and then dividing it into 2n equal parts – so four parts for a circle, eight for a 3D sphere, 16 for a 4D sphere and so on. The researchers then mathematically stretch and squeeze these segments to alter their shape without changing their width. “The recipe is very simple, but we understood that only after all of our elaboration,” says team member Andriy Bondarenko at the Norwegian University of Science and Technology.
The team proved that it is always possible to do this distortion in such a way that you end up with a shape that has a volume at most 0.9n times that of the equivalent dimensional sphere. This means that as you move to higher and higher dimensions, the shape of constant width gets proportionally smaller and smaller compared with the sphere.
Visualising this is difficult, but one trick is to imagine the lower-dimensional silhouette of a higher-dimensional object. When viewed at certain angles, the 3D shape appears as a 2D Reuleaux triangle (see the middle image above). In the same way, the 3D shape can be seen as a “shadow” of the 4D one, and so on. “The shapes in higher dimensions will be in a certain sense similar, but will grow in complexity as [the] dimension grows,” says Arman.
Having identified these shapes, mathematicians now hope to study them further. “Even with the new result, which takes away some of the mystery about them, they are very mysterious sets in high dimensions,” says Kalai.
For more such insights, log into www.international-maths-challenge.com.
*Credit for article given to Alex Wilkins*