Some students get their first look at manipulating equations when they learn how to convert a repeating decimal to its corresponding fraction (rational numbers yield terminating decimals, or non-terminating repeating decimals). Playing with fractions and decimals is always good in itself, but there are also two nice side-benefits to this simple calculation: first, you can see the surprising result that 0.999… = 1, and second, it helps answer the question ‘which fractions lead to terminating decimals, and which lead to repeating decimals?’ Better still, it leads to other questions.
- The calculation – turning decimals into fractions
A repeating decimal like 0.633633… can be written as a fraction by writing out the equation x = 0.633633…, and then manipulating it so that the infinitely long repeated parts get eliminated. The manipulation is done by multiplying the equation by the right power of 10 to shift the digits, and then subtracting off the original equation.
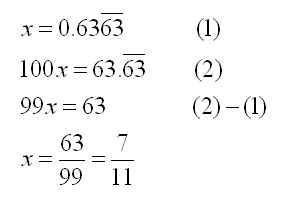
Perhaps you have a more complicated repeating decimal that you want to fractionalize. The procedure remains essentially the same: obtain two equations that have the same “decimal part” that can be eliminated by subtracting. You might have to shift twice in order to accomplish this. Here is an example:
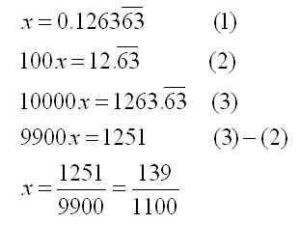
- Showing that 0.999… = 1
This school mathematics result has been made into a popular topic of discussion on the Internet. Try doing a search on “0.999 = 1”, and see what you find.
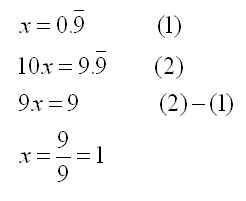
- Which fractions terminate, and which keep going?
A fraction can sometimes give us a terminating decimal, and sometimes a repeating decimal. It is reasonable to wonder what kind of fractions lead to each kind of decimal.
Thinking about the procedure above might give you an idea for how to think about this question.
If we have a situation where a fraction x = p/q is a terminating decimal, it means that there must be some power of 10, say 10^n, that if we multiply x by this power we can completely eliminate the decimal (i.e. multiplying by 10^n gives us an integer). This could only happen if the denominator q divides 10^n. This would require q to be of the form (2^m)(5^k), where the exponents m and k are non negative integers (possibly zero), and n = max(m, k).
So, a fraction p/q terminates if and only if the denominator is of the form (2^m)(5^k). Consequently, fractions such as 1/2, 3/5, 9/25, 7/8, 3/200, are all terminating, and, for example, any fraction of the form 1/p where p is a prime that is not 2 or 5, will be non-terminating and repeating.
It is reasonable to ask questions about how long the decimals or their repeating parts can be. For a non-terminating repeating decimal, questions about the length of the repeating part are harder to answer.
For more such insights, log into www.international-maths-challenge.com.
*Credit for article given to dan.mackinnon*

